In this article, we will learn how to use Bitwise operators and some tricks to deal with our common problems. Let’s get started.

Table of Contents

• Introduction to Bitwise operators
• When to use
• Benefits and Drawbacks
• Some tricks in Bit Manipulation
• Wrapping up

Introduction to Bitwise operators

Bitwise operators are operators that perform with each bit of a number. But we can use them with some integral types such as char, short, int, …

There are some operators:

• Bitwise AND &
• Bitwise OR |
• Bitwise XOR ^
• Bitwise Complement ~
• Shift operator >> or <<

1. Bitwise AND &

    1 & 1 = 1
    1 & 0 = 0
    0 & 0 = 0
    0 & 1 = 0
   

   So if both bits are 1, then AND operator gives 1, otherwise 0.

2. Bitwise OR |

    1 | 1 = 1
    1 | 0 = 1
    0 | 1 = 1
    0 | 0 = 0
   

   If either of bits are 1, then OR operator gives 1, otherwise 0.

3. Bitwise XOR ^

    1 ^ 1 = 0
    1 ^ 0 = 1
    0 ^ 1 = 1
    0 ^ 0 = 0
   

   When performing XOR on two integers, the XOR operation is calculated on each pair of bits (the two bits at the same index in each number).

    5 ^ 6  // gives 3
   
    // At the bit level:
    //     0101  (5)
    //   ^ 0110  (6)
    //   = 0011  (3)
   

4. Bitwise Complement ~

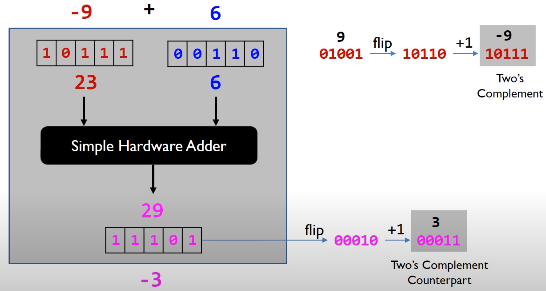
    ~5 = ~0101 = 1010
   

   This operator will return the one’s complement representation of the input value by inversing all bits in a variable, 1 to 0, or 0 to 1.

   Then the highest bit is 1, the complier or OS will give the two’s complement for this number. It looks like that the below image.

    ~5 = 1010 (binary) = 10 (decimal)  // (the one's complement)
    -6 = ~0110 = 1001 --> (+1) --> 1010
   

   The two’s complement simplifies the hardware implementation of arithmetic functions.

   • For addition or substraction in two’s complement, the hardware can ignore the sign of operands if signed integers are represented.
   • For multiplication, the multiplication hardware can also ignore the sign of operands if the product is required to keep the same number of bits as operands.

   –> This property simplifies the hardware design for addition, substraction, and multiplication. This property does not hold true for division.

   

5. Shift operator

   A bit shift moves each digit in a number’s binary representation left or right. There are three main types of shifts:

   • Left shifts

     When shifting left, the most-significant bit is lost, and a 0 bit is inserted on the other end.

     The left shift operator is usually written as “«”.

       0010 << 1  →  0100
       0010 << 2  →  1000
     

     A single left shift multiplies a binary number by 2:

       0010 << 1  →  0100
     
       0010 is 2
       0100 is 4
     

   • Logical Right shifts

     When shifting right with a logical right shift, the least-significant bit is lost and a 0 is inserted on the other end.

       1011 >>> 1  →  0101
       1011 >>> 3  →  0001
     

     For positive numbers, a single logical right shift divides a number by 2, throwing out any remainders.

       0101 >>> 1  →  0010
     
       0101 is 5
       0010 is 2
     

   • Arithmetic Right shifts

     When shifting right with an arithmetic right shift, the least-significant bit is lost and the most-significant bit is copied.

     Languages handle arithmetic and logical right shifting in different ways. Java provides two right shift operators: » does an arithmetic right shift and »> does a logical right shift.

       1011 >> 1  →  1101
       1011 >> 3  →  1111
     
       0011 >> 1  →  0001
       0011 >> 2  →  0000
     

     The first two numbers had a 11 as the most significant bit, so more 11’s were inserted during the shift. The last two numbers had a 0 as the most significant bit, so the shift inserted more 0’s.

     If a number is encoded using two’s complement, then an arithmetic right shift preserves the number’s sign, while a logical right shift makes the number positive.

       // Arithmetic shift
       1011 >> 1  →  1101
           1011 is -5
           1101 is -3
     
       // Logical shift
       1111 >>> 1  →  0111
           1111 is -1
           0111 is  7
     

When to use

• when performing update and query operations of Binary Indexed Tree.

Benefits and Drawbacks

1. Benefits

   • fast.

2. Drawbacks

   • It’s difficult to understand, read code, maintain code.

Some tricks in Bit Manipulation

1. Clear all bits from LSB to ith bit

    mask = ~((1 << (i + 1) - 1);
    x &= mask
   

2. Clear all bits from MSB to ith bit

    mask = (1 << i) - 1;
    x &= mask;
   

3. Check whether a character is upper case

    char ch = 'A';
    boolean isUpperCase = (ch & 32) == 0;
   

   In ASCII, the distance between upper cases and lower cases is 32. So, if a character is upper case, it does not contain 5th bit –> 0010 0000.

4. Convert upper case to lower case

    char ch = 'A';
    char lowerCase = ch | 32;
   

5. Convert lower case to upper case

    int distance = 32;
    char ch = 'a';
    char upperCase = ch & ~distance; // we get all characters that excepts 5th bit.
   

Wrapping up

• Understanding the meaning of all basic bitwise operators.

Refer:

https://www.geeksforgeeks.org/bits-manipulation-important-tactics/

https://www.vojtechruzicka.com/bit-manipulation-java-bitwise-bit-shift-operations/

https://www.log2base2.com/C/bitwise/bitwise-ones-complement-operator-in-c.html

https://www.interviewcake.com/concept/java/bit-shift